A Mathematical Review A.3 Affine transforms A.5 Spatial velocities and forces

A.4 Rotational velocity

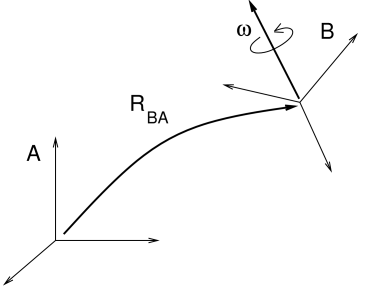
Figure A.8: Frame B rotating with respect to frame A.

Given two 3D coordinate frames A and B, the rotational, or angular, velocity of B with respect to A is given by a 3D vector $\boldsymbol{\omega}_{BA}$ (Figure A.8). $\boldsymbol{\omega}_{BA}$ is related to the derivative of ${\bf R}_{BA}$ by

$\dot{\bf R}_{BA}=[{}^{A}\boldsymbol{\omega}_{BA}]{\bf R}_{BA}={\bf R}_{BA}[{}^% {B}\boldsymbol{\omega}_{BA}]$

(A.21)

where ${}^{A}\boldsymbol{\omega}_{BA}$ and ${}^{B}\boldsymbol{\omega}_{BA}$ indicate $\boldsymbol{\omega}_{BA}$ with respect to frames and and $[\boldsymbol{\omega}]$ denotes the $3\times 3$ cross product matrix

$[\boldsymbol{\omega}]\equiv\left(\begin{matrix}0&-\omega_{z}&\omega_{y}\\ \omega_{z}&0&-\omega_{x}\\ -\omega_{y}&\omega_{x}&0\\ \end{matrix}\right).$

(A.22)

If we consider instead the velocity of with respect to , it is straightforward to show that

$\boldsymbol{\omega}_{AB}=-\boldsymbol{\omega}_{BA}.$

(A.23)

A.3 Affine transforms Bibliography A.5 Spatial velocities and forces

Generated on Sun Jan 12 23:00:57 2025 by LaTeXML [LOGO]